Definition of MAP

In general, a MAP is a point process defined by the epochs of some transitions in a Markov chain.

Definition 15   Consider a Markov chain with infinitesimal generator $\mathbf{D}=\mathbf{D}_0+\mathbf{D}_1$, where all the off-diagonal elements of $\mathbf{D}_0$ and all the elements of $\mathbf{D}_1$ are nonnegative. The transitions associated with $\mathbf{D}_1$ are called type 1 transitions. A MAP with parameters ( $\mathbf{D}_0,\mathbf{D}_1$), MAP( $\mathbf{D}_0,\mathbf{D}_1$), is a point process where an event occurs when a type 1 transition occurs in the Markov chain.

Figure 3.11 illustrates a MAP of order 2 having parameters

$$\mathbf{D}_0 = \left(\begin{array}{cc} -\sigma_1 & \alpha_{1... ...ambda_{21} \\ \lambda_{21} & \lambda_{22} \end{array}\right),$$

where $\sigma_i = \sum_{j\neq i} \alpha_{ij} + \sum_j \lambda_{ij}$ for $i=1,2$..

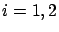Figure 3.11: A MAP(2). Transitions shown in thick arrows (transitions with $\lambda_{ij}$) are associated with events in the MAP(2). Transitions with $\alpha_{ij}$ only change the state.

To completely specify a MAP, the initial probability vector in the Markov chain needs to be specified. Throughout, we assume that the initial probability vector is the same as the stationary probability vector. That is, our MAPs are stationary MAPs.

A MAP( $\mathbf{D}_0,\mathbf{D}_1$) is called a Markov modulated Poisson process, MMPP, if $\mathbf{D}_1$ is diagonal. That is, in the Markov chain that defines an MMPP, all the transitions that are associated with events do not change the state.