


Next: Bibliography
Up: Appendix A
Previous: Proof of Theorem 6
 becomes in that case
becomes in that case
where
![$\Delta=\vec{v}[1] - \vec{v}[0]$](img607.png) . There are five different values for
. There are five different values for 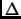 , giving rise to nine different
, giving rise to nine different  :
:
Fix 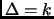 where
where
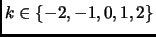 .
.
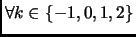 , the value
, the value 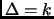 should be preferred to the value
should be preferred to the value  iff the corresponding
iff the corresponding  is smaller, that is :
is smaller, that is :
Rearranging terms gives
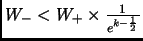 . This leads to the rule of the lemma.
. This leads to the rule of the lemma.
©2002 AI Access Foundation and Morgan Kaufmann Publishers. All rights reserved.
![]() becomes in that case
becomes in that case
